Abstract

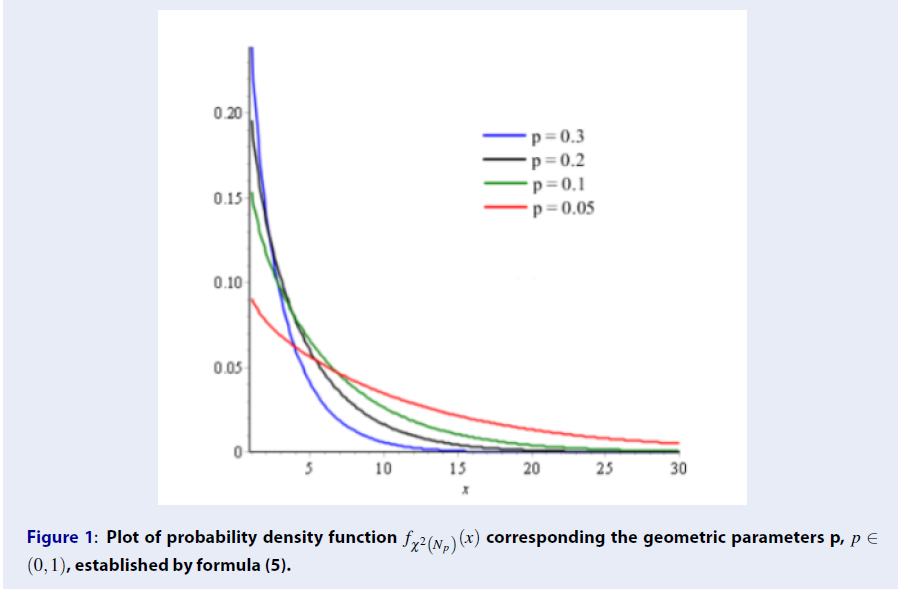
The chi-square distribution with degrees of freedom has an important role in probability, statistics and various applied fields as a special probability distribution. This paper concerns the relations between geometric random sums and chi-square type distributions whose degrees of freedom are geometric random variables. Some characterizations of chi-square type random variables with geometric degrees of freedom are calculated. Moreover, several weak limit theorems for the sequences of chi-square type random variables with geometric random degrees of freedom are established via asymptotic behaviors of normalized geometric random sums.