Homology of Borel Subgroup of SL(2,\mathbb{F}_p)

Abstract

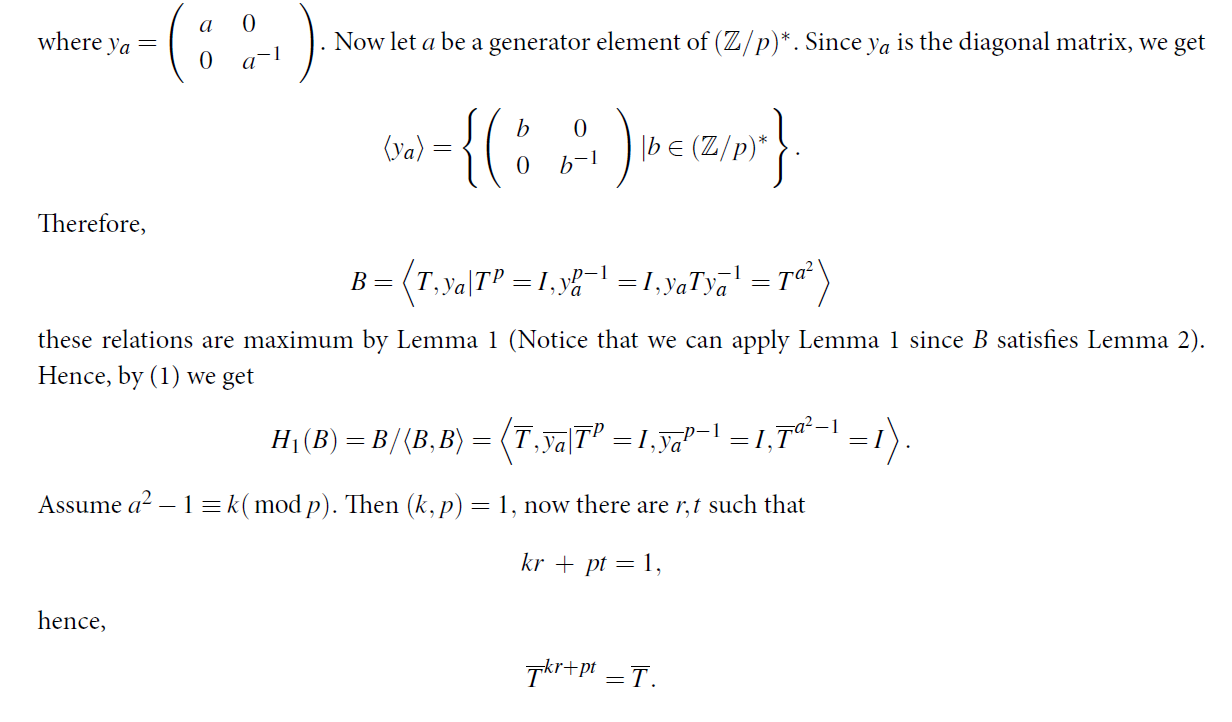
In this paper we compute the integral homology of the Borel subgroup $B$ of the special linear group $SL(2,\mathbb{F}_p), p$ is a prime number. Arcoding to Adem \cite{AJM} these are periodic groups. In order to compute the integral homology of $B,$ we decompose it into $\ell-$ primary parts. We compute the first summand based on Invariant Theory and compute the rest summand based on Lyndon-Hochschild-Serre spectral sequence. We assume that $p$ is an odd prime and larger than $3.$