A brief introduction to Quillen conjecture

Abstract

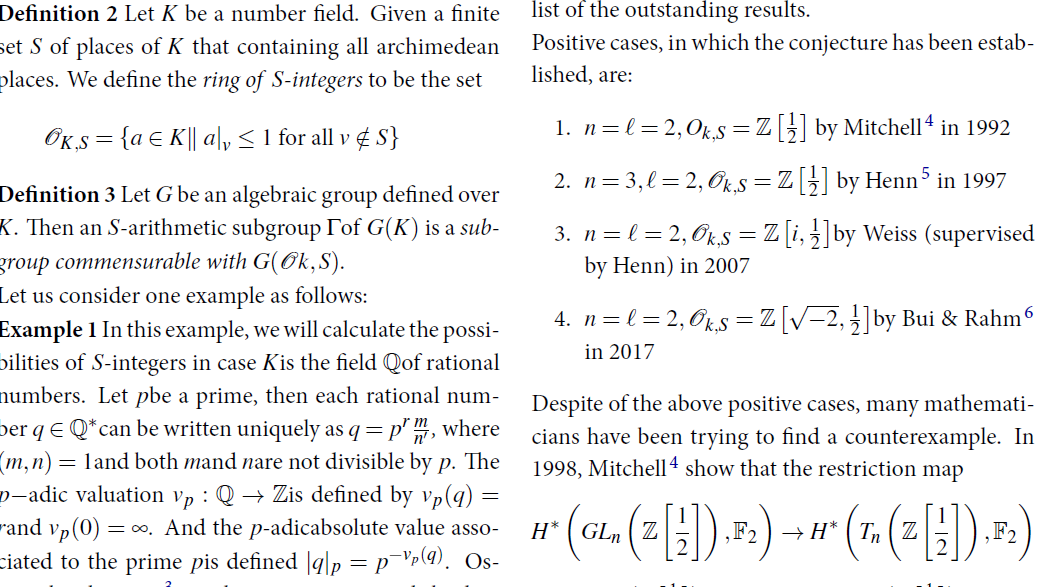
Introduction: In 1971, Quillen stated a conjecture that on cohomology of arithmetic groups, a certain module structure over the Chern classes of the containing general linear group is free. Over time, many efforts has been dedicated into this conjecture. Some verified its correctness, some disproved it. So, the original Quillens conjecture is not correct. However, this conjecture still has great impacts on the field cohomology of group, especially on cohomology of arithmetic groups. This paper is meant to give a brief survey on Quillen conjecture and finally present a recent result that this conjecture has been verified by the authors.

Method: In this work, we investigate the key reasons that makes Quillen conjecture fails. We review two of the reasons: 1) the injectivity of the restriction map; 2) the non-free of the image of the Quillen homomorphism. Those two reasons play important roles in the study of the correctness of Quillen conjecture.

Results: In section 4, we present the cohomology of ring ​ which is isomorphic to the free module ​ over ​. This confirms the Quillen conjecture.

Conclusion: The scope of the conjecture is not correct in Quillens original statement. It has been disproved in many examples and also been proved in many cases. Then determining the conjectures correct range of validity still in need. The result in section 4 is one of the confirmation of the validity of the conjecture.